Premise

Since sound is a wave, it can have a property that measures the quantity of energy transported by waves when they pass through a section of unit area in a unit of time. It is measured in ${\displaystyle {\rm {W/m^{2}}}}$. In the simple case of sine waves (or of pure sounds), it is proportional to the square of the amplitude of the wave oscillation. This property is called sound intensity. On the level of perception, it is of importance (albeit not exclusively) in describing what we presently call sound volume or loudness. In music, this is known as dynamics; i.e. those indications on sheet music that range from ${\displaystyle ppp\;}$ ("pianissimo") to ${\displaystyle fff\;}$ ("fortissimo").

Audible intensities

Threshold of audibility

The threshold of audibility is defined as the minimum intensity ${\displaystyle I_{\mathrm {min} }\;}$ that the human ear is able to perceive. Experience shows us that this threshold varies from person to person (for example, it rises as a subject ages) and, above all, depends on the frequency of the sound heard.

Generally, a standard value is used, which has been obtained by averaging the threshold of audibility of many individuals for a pure sound with a frequency of 1000 Hz.

The value of this threshold is extremely small and equals

${\displaystyle I_{\rm {min}}=10^{-12}{\rm {W/m^{2}}}\;}$

and corresponds to a variation of pressure with respect to atmospheric pressure in the absence of sound of only 20 ${\displaystyle \mu }$Pa (equal to about 0.2 billionths of atmospheric pressure).

The pain threshold

At the other end of the range of audible intensities, we have the pain threshold, or the maximum intensity that the human ear can perceive and beyond which sound becomes a feeling of pain (however, it has been observed that sound can permanently damage hearing even at lower intensities depending on the conditions of exposure). This value is equal to

${\displaystyle I_{\rm {max}}=1{\rm {W/m^{2}}}\;}$

and is a thousand billion times (${\displaystyle 10^{12}}$) larger than the threshold of audibility.

Sound intensity levels in decibels (SIL dB)

As shown in the previous paragraph, the range of variation of intensity is extremely vast and covers about 12 orders of magnitude. If it were compared to a scale of lengths, it would range from the size of an amoeba (about 600 thousandths of a mm) to the diameter of the lunar orbit (about 600 thousand km).

This massive variability, along with the fact that the ear is sensitive to pressure variations and not the absolute value of the pressure itself, determined the choice to express the measurement of intensity with a logarithmic scale.

Therefore, sound intensity level (SIL) is defined as

${\displaystyle I({\rm {dB_{SIL}}})=10\log _{10}\left({\frac {I}{I_{\rm {min}}}}\right)}$

(1)

where

${\displaystyle I_{\rm {min}}=10^{-12}{\rm {W/m^{2}}}\;}$

(2)

.

Sound intensity level is a pure number (adimensional quantity), to which is attributed a conventionally agreed unit of measurement: the decibel (from A.G. Bell, a scientist from the United States of America) with the symbol "dB". The decibel is a unit of measurement that does not belong to the International System and is derived from the relationship between sound intensity and the threshold of audibility.

Advantages of the decibel scale

Measuring the sound intensity level that reaches the ear with a logarithmic scale related to the threshold of audibility, rather than with the absolute value of sound energy (or pressure), presents a series of advantages:

• the value of intensity relative to the threshold of audibility is exactly 0 dB

in fact, if we replace I with the value ${\displaystyle I_{\rm {min}}=10^{-12}{\rm {W/m^{2}}}}$ we obviously get

${\displaystyle I_{\rm {min}}^{\rm {(dB)}}=10\log _{10}\left({\frac {I_{\rm {min}}}{I_{\rm {min}}}}\right)=10\cdot \log _{10}1=0\ {\rm {dB}}}$

• the opportunity to represent properties with a large range of variation

in the case of sound, the range of variation is incredibly vast: the sound level of a rock concert (near the pain threshold; and that's not just a question of taste!) is 1000 billion times more intense than the threshold of audibility. Using the definition of ${\displaystyle I^{\rm {(dB)}}}$, if we replace I with I_max, we get:

${\displaystyle I_{\rm {max}}^{\rm {(dB)}}=10\log _{10}\left({\frac {10^{12}I_{\rm {min}}}{I_{\rm {min}}}}\right)=10\cdot \log _{10}10^{12}=120\ {\rm {dB}}}$

• On the level of perception, the ear seems to approximately respond to the intensity of a sound stimulus according to a logarithmic scale.

perceived volume (loudness) is not in linear relation to sound intensity. In other words, if sound intensity is doubled (this can be done objectively in a laboratory), a sound of twice the loudness is not perceived (the subjectivity of various listeners comes into play here). Repeated experiments conducted on various listeners have shown that to obtain sounds of double the perceived loudness, the intensity of the sound wave must be increased by approximately a factor of ten.

progressione_aritmetica_ampiezze.wav A pure sound of growing amplitude with arithmetic progression: the amplitude increases every second by a fixed quantity (equal to the initial amplitude)

progressione_geometrica_ampiezze.wav A pure sound of growing amplitude with geometric progression: the amplitude doubles every second

• this scale presents the "right" level of sensitivity

this is obtained by multiplying the logarithm of the intensity ratio in definition (1) by a factor of 10. This factor is necessary, since without it we would have obtained a unit of measurement called the Bel, which is ten times greater than the decibel. One decibel is, with good approximation, the minimum difference in the intensity between two sounds that the ear can perceive (that which is called in specialised literature the JND, an acronym for Just Noticeable Difference). This means that the ear notices a loudness difference between two sounds only if their intensity levels differ by more than one decibel. We talk about the right level of sensitivity in this sense. In fact, what is the use of having unit of measurements smaller than decibels if the ear cannot appreciate the difference? It would be like having a ruler showing tenths of a millimetre: our eye would not be able to perceive the difference between two consecutive "marks".

• For other curiosities and questions about the decibel scale, see the section on questions and answers

Intensity levels of typical sounds

The following table presents the relative levels of intensity in a logarithmic scale, i.e. in decibels, in relation to the standard intensity of just perceivable sound. These values are associated with several situations from everyday life (to see a similar table showing sound pressure levels corresponding to these intensities, visit the page on sound pressure levels).

Sorgente sonora	Livello di intensità sonora in dB
pain threashold	134
ear damages in the short term	ca. 120
Jet at 100 m	110 - 140
pneumatic hammer at 1 m / Disco	ca. 100
ear damages in the long term	ca. 90
heavy traffic road at 10 m	80 - 90
car at 10 m	60 - 80
TV at 1 m	ca. 60
talking at 1 m	40 - 60
very quiet room	20 - 30
breath	10
threshold of audibility at 2 kHz	0

• A sound intensity that ranges from 0 dB to 60 dB is considered quiet.
• A sound intensity that ranges from 60 dB to 90 dB (such as car traffic) can be dangerous, particularly if it continues over time.
• A sound intensity that ranges from 90 dB to 120 dB (such as in discotheques, at construction sites, in airports or near exploding firecrackers) can be very damaging.
• It is believed that a noise of 200 dB could kill a person.
• These values are simply indicative. More important to the studying of acoustic pollution, is the measurement of intensity in octave bands; i.e. in fixed frequency intervals.

To see data on the loudest sounds ever produced, see the paragraph on What is the loudest possible sound that can be produced?.

Perceived loudness

Sound intensity ${\displaystyle I^{\mathrm {(dB)} }}$ is a property that objectively measures the flow of energy transported by a sound wave. However, this property does not correctly describe perceived loudness because it strongly depends on sound frequency, and in lesser measure, on the timbre of the sound itself. A spectacular example of this can be experienced by listening (with headphones) to this continuously increasing sound frequency (it goes from 20 Hz to 20000 Hz). The objective sound intensity is constant, as can be seen in the image of the envelope of the wave form shown next to the sound.

sweep_20_20000_Hz.wav Sound of constant intensity and increasing frequency. Perceived loudness seems variable partly due to the response of the device with which the sound is transmitted (headphones, speakers, etc.) and partly due to the dependence on frequency of the equal-loudness contours (see text)

This experiment leaves no doubts:

• perceived loudness greatly increases for the central frequencies (you would have been forced to lower the volume to bear the sound of such frequencies);
• if you adjust the volume to "bear" the sound of such frequencies and listen again, you will observe that the high frequencies, and even more so the low frequencies, become almost inaudible.

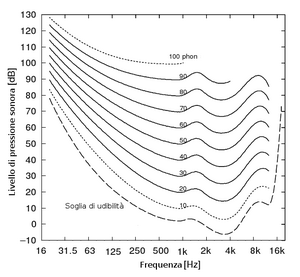
Therefore, perceived loudness presents a complex link to sound intensity. To adequately describe it, we decided to use equal-loudness contours that show, along with the frequency variation, the locus of the points for which perceived loudness is constant. These have been obtained by asking the listener, while listening to sounds of various frequencies, to adjust the volume knob in such a way as to perceive them with the same loudness. The curves obtained, which is not completely unexpected if you have understood the sense of the previous sound experiment, are illustrated in the figure for the various values of perceived loudness (represented by the number above the curves):

We can draw several significant conclusions from the graph:

• the level of perceived loudness is conventionally fixed as equal to the level of loudness ${\displaystyle I^{\rm {(dB)}}}$ at the frequency of 1000 Hz.
• if the perceived loudness were completely determined only by the loudness of the source, the equal-loudness contours would be horizontal. This is fairly true for frequencies that range from 200 Hz to 6000 Hz (which are also the most important for making music);
• the ear's sensitivity is reduced at low and high frequencies. To perceive a sound with a frequency of 50 Hz at the intensity of 10 dB you need an intensity ${\displaystyle I^{\rm {(dB)}}}$ of 60 dB (about 1000000 times more!). One can see that by following the 10 dB equal-loudness contour and reading the value of abscissa corresponding to 50 Hz. In the same way, which was already shown in a previous paragraph, to hear a sound at 20 Hz, the threshold of audibility rises to 70 dB (about 10 million times more than the threshold at 1000 Hz);
• following a horizontal line (for example, at a loudness of I^(dB)=70 dB) until it meets the equal-loudness curve at 40 dB, we realise that this intersection is at about the frequencies of 100 and 15000 Hz. At those frequencies, perceived loudness is a thousand times (from 70 dB to 40 dB) smaller than that perceived at 1000 Hz. This explains the drastic drop in perceived loudness that occurred at low and high frequencies in the sound experiment;
• observing the curves, we notice the presence of a minimum (i.e. a maximum sensitivity) at a frequency just below 4000 Hz; this frequency corresponds to the resonance frequency of the auditory canal (for more details see the page on the anatomy of the auditory system). The next resonance frequency (three times the fundamental because the canal is equivalent to a closed bore) is about 12000 Hz and creates around this frequency the "bending" that explains the "slowing" of the loss of sensitivity at high frequencies.

rumore_bianco_intens_crescente.wav In this example, you can listen to a hiss called white noise, which contains all the frequencies of the audible spectrum. It has an increasing loudness at a rate of 3 dB per second. This means that the signal intensity doubles every second.

Another unit of measurement: the phon

In the previous paragraph, we measured the perceived loudness and the level of sound intensity with the same unit of measurement, the decibel. Since the two properties are coincident only at the conventional frequency level of 1000 Hz, it was decided to introduce a new unit of measurement, the phon, to measure perceived loudness. The usefulness of this differentiation is in the fact that it allows us to immediately understand if we are talking about intensity (in decibels) or perceived loudness (in phons). For example, we can say that, reformulating one of the examples of the equal-loudness contours, a sound at 70 dB is perceived at a frequency of 100 Hz at 40 phons. This way, there is no more need to clarify that 70 dB refers to sound intensity and 40 phons to perceived loudness: this is now obvious in the definition of phon!

In-depth study and links

• If you are interested in knowing through which "mechanisms" our auditory system achieves its extraordinary sensitivity (indicated by the low value of the threshold of audibility), visit the page on the anatomy of the auditory system.
• On the page about useful decibel values, you will find a useful table for converting the ratio of two intensities I/I_min into I^(dB).
• In the questions and answers section, you will find a lot of information on the measurement of both the objective intensity and the perception of a sound.

---